What is the Boer-Mulders function or effect?
The formal definition of the Boer-Mulders (BM) function was given in the publication: D.
Boer, P.J. Mulders, "Time reversal odd distribution functions in leptoproduction,'' Physical Review D 57 (1998) 5780, preprint version (november 1997)
For those familiar with Quantum Field Theory language, it is given by:
The state |P> denotes a proton with a momentum P and the right hand
side is the expection value of a specific quark operator inside the
proton. This quark operator is nonlocal and contains a Wilson line L that makes it color gauge invariant. The Dirac structure projects out a
specific quark polarization state (a helicity flip state or
transversely polarized state). F.T. denotes a three-dimensional Fourier
transform and C is a specific path of the Wilson line L that depends on the process in a calculable and therefore predictable way.
What this quantity reflects is the net polarization in direction i
carried by the quarks inside an unpolarized proton. Protons and quarks
are both spin-1/2 particles that can be in certain polarization states
(spin up or down in a specific direction). For an unpolarized proton
these states are averaged over. It turns out that the quarks can be
polarized on average even inside such an unpolarized proton, as long as
they are not moving collinearly. The quarks inside a proton as seen by
a highly relativistic probe are generally not moving in exactly the
same direction as the proton. If the proton moves along the z direction
(or is viewed along the z direction), then the quarks can have some
transverse momentum kT with respect to the proton momentum,
which together define a plane. If the above function is nonzero, it
means there is a net quark polarization orthogonal to that plane.
Pictorially this can be displayed like:
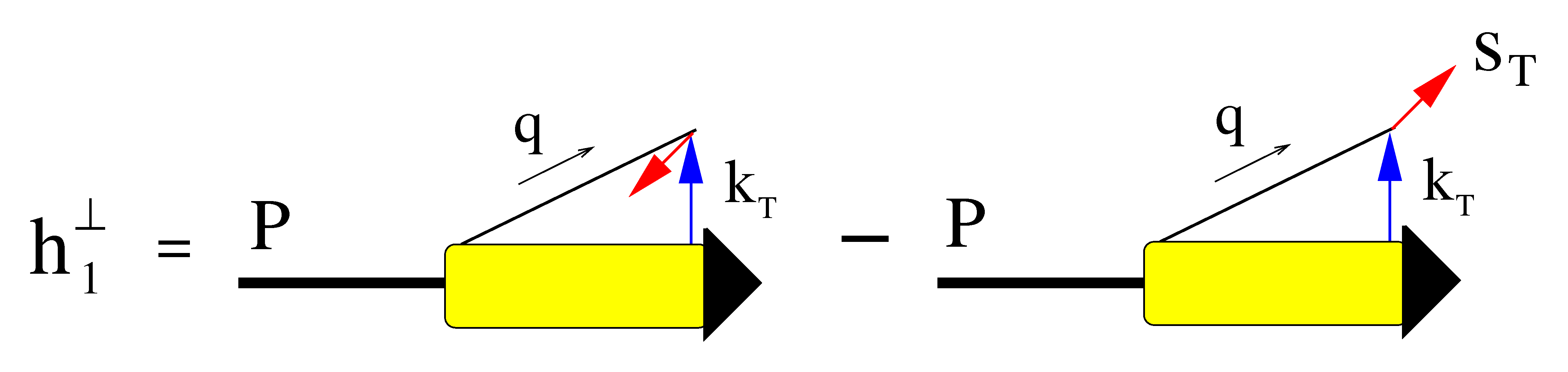
It reflects the presence of a handedness inside the proton (P.(kT x sT)),
albeit one that is process dependent. It really requires a process in
order for it to make sense. But that is true for any quark
distribution: only when one views the proton with a sufficiently
energetic probe, one can speak of quark distributions inside the proton
to begin with. So the BM function is a quark distribution that quantifies a particular
spin-orbit correlation. It is similar to the Sivers function (D.
Sivers, "Single spin production asymmetries from the hard scattering of
point-like constituents", Physical Review D 41 (1990) 83) which refers to unpolarized quarks inside a transversely polarized proton.
Is it a property of the proton?
It requires a proton (or any other hadron for that matter) and
interactions with that proton. In a sense it is a property of the
proton "in action", so it is not a static property (a part of the
proton wave function), but rather a dynamic one. This does not make it
less fundamental, in my opinion. This is simply how one sees the proton
in high energy scattering processes. I like to compare it to an X-ray
picture of myself which displays what I look like when viewed by
X-rays. The X-rays are not part of me, but their interaction with me
does produce a picture of me, even if it looks different from the more
familiar ones in the visible light spectrum.
Is there any evidence that this function is nonzero?
Yes, there is a first-principles demonstration using lattice QCD: B.U. Musch et al., "Sivers and Boer-Mulders observables from lattice QCD", Physical Review D 85 (2012) 094510
Furthermore, this distribution can offer an explanation for an
anomalously large asymmetry in the Drell-Yan process which was measured in 1986
and 1989 independently at CERN and Fermilab. I showed this in the
article: D. Boer, "Investigating the origins of transverse spin asymmetries at RHIC,'' Physical Review D 60 (1999) 014012 Currently (september 2014) there are experimental investigations ongoing that may provide further evidence for this effect.
In addition, it is allowed by all the symmetries of the theory (QCD), so there is no reason why it would have to be zero.
Is it related to the electric dipole moment?
No, the electric dipole moment (EDM) operator of quarks is a local operator,
the one one would get by integrating over all quark momenta, which for
the BM function gives identically zero (as it is an odd function of kT).
Moreover, the BM function is parity conserving, whereas the EDM violates P and CP. It is also not related
to the EDM of the proton which is the expectation value of a (local)
vector operator, not of a tensor operator.
Rather, the BM function may be related to a kind of anomalous magnetic moment, see M.
Burkardt, "Transverse deformation of parton distributions and
transversity decomposition of angular momentum,'' Physical Review D 72
(2005) 094020
Some statistics (september 2014)
There are currently 20 research papers by other people that have Boer-Mulders in the title
The original paper is currently cited more than 600 times in the Inspire HEP database and around 500 times in the Web of Science database